njtynhj

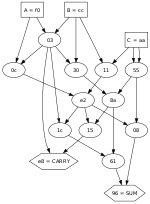
或非门全加器

或非门（英语：NOR gate）是数字逻辑中实现逻辑或非的逻辑门，功能见右侧真值表。若输入均为低电平（0），则输出为高电平（1）；若输入中至少有一个为高电平（1），则输出为低电平（0）。或非是逻辑或加逻辑非得到的结果。或非是一种具有函数完备性的运算，因此其他任何逻辑函数都能用或非门实现。相比之下，逻辑或运算器是一种单调的运算器，其只能将低电平变为高电平，但不能将高电平变为低电平。

在绝大多数但不是所有的电路设计中，逻辑非的功能本身就包含在结构中，如CMOS和TTL等。在这样的逻辑系列中，要实现或门，唯一的方法是用2个或更多的逻辑门来实现，如一个或非门加一个反相器，但一个重要的例外是多米诺逻辑（英语：domino logic），因为其结构中本身就没有反相逻辑。

概述

下列包括逻辑门的3种符号：形状特征型符号（ANSI/IEEE Std 91-1984）、IEC矩形国标符号（IEC 60617-12）和不再使用的DIN符号（DIN 40700）。其他的逻辑门符号见逻辑门符号表。

表达式	符号			功能表	继电器逻辑
	ANSI/IEEE Std 91-1984	IEC 60617-12	DIN 40700
Y=A+B¯displaystyle Y=overline A+B Y=A∨B¯displaystyle Y=overline Avee B Y=A∨¯Bdisplaystyle Y=Aoverline vee B			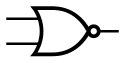	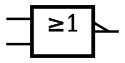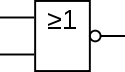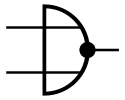A B Y=A+Bdisplaystyle Y=A+B, Y=A+B¯displaystyle Y=overline A+B 0 0 0 1 0 1 1 0 1 0 1 0 1 1 1 0

硬件描述和引脚分配

或非门是基本的门电路，因此常用於晶体管－晶体管逻辑（TTL）和CMOS集成电路。标准4000系列CMOS集成电路是4001，包含四个2输入或非门。引脚分配如下：

4001四或非门DIP封装集成电路的引脚分配图

.mw-parser-output .templatequotemargin-top:0;overflow:hidden.mw-parser-output .templatequote .templatequoteciteline-height:1em;text-align:left;padding-left:2em;margin-top:0.mw-parser-output .templatequote .templatequotecite citefont-size:small 输入A1 输入B1 输出Q1 输出Q2 输入B2 输入A2 VSS 输入A3 输入B3 输出Q3 输出Q4 输入B4 输入A4 VDD

可用型号

大多数半导体制造商都生产这种元件，如飞兆半导体公司、飞利浦、德州仪器，封装方式分为直插DIP封装和SOIC封装（英语：small-outline integrated circuit）两种。元件的数据表可在大多数元件数据库查询到。

常用的CMOS和TTL逻辑系列（英语：logic family）中，也有8输入或非门：

• 
  CMOS
  
  • 4001：四2输入或非门
  
  
  • 4025：三3输入或非门
  
  
  • 4002：双4输入或非门
  
  
  • 4078：单8输入或非门
  
  


• 
  TTL
  
  • 7402：四2输入或非门
  
  
  • 7427：三3输入或非门
  
  
  • 7425：双4输入或非门（有闸门，已不再使用）
  
  
  • 74260：双5输入或非门
  
  
  • 744078：单8输入或非门
  
  

或非门在老式的RTL和ECL系列中很高效，使用非常普遍。

实现

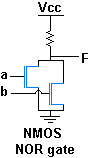CMOS或非门的集成电路版图

上图展示了使用NMOS线路的2输入或非门的构造。如果输入都是高电平，对应的NMOS就会接通，输出会被拉到低电平；反之输出会通过上拉电阻被拉到高电平。下图展示了使用CMOS技术的2输入或非门。输出端的二极管和电阻用来保护CMOS元件，以防其受到静电放电（ESD）的损害，从而在电路的逻辑功能中发挥不了作用。

无缓冲CMOS 2输入或非门

备选方案

与非门构建的或非门

或非门构建的与非门

如果没有专门的或非门，可以用与非门实现或非门，方法是将两个输入接在一起的与非门作为另外一个与非门的两个输入，然後在後者的输出接一个输入接在一起的与非门，即可实现或非门。任何逻辑门都可以用与非门的组合或或非门的组合实现。

或非逻辑

与非门实现的或非门逻辑函数如下：

x∨¯y=[(x∧¯x)∧¯(y∧¯y)]∧¯[(x∧¯x)∧¯(y∧¯y)]displaystyle xoverline lor y=left[left(xoverline land xright)overline land left(yoverline land yright)right]overline land left[left(xoverline land xright)overline land left(yoverline land yright)right]

或非门具有函数完备性，和与非门一样可以仅用其实现其他所有的逻辑功能。

NOT（非）	x¯displaystyle overline x	≡displaystyle equiv	x∨¯xdisplaystyle xoverline lor x
AND（与）	x∧ydisplaystyle xland y	≡displaystyle equiv	(x∨¯x)∨¯(y∨¯y)displaystyle left(xoverline lor xright)overline lor left(yoverline lor yright)
NAND（与非）	x∧¯ydisplaystyle xoverline land y	≡displaystyle equiv	[(x∨¯x)∨¯(y∨¯y)]∨¯[(x∨¯x)∨¯(y∨¯y)]displaystyle left[left(xoverline lor xright)overline lor left(yoverline lor yright)right]overline lor left[left(xoverline lor xright)overline lor left(yoverline lor yright)right]
OR（或）	x∨ydisplaystyle xlor y	≡displaystyle equiv	(x∨¯y)∨¯(x∨¯y)displaystyle left(xoverline lor yright)overline lor left(xoverline lor yright)
NOR（或非）	x∨¯ydisplaystyle xoverline lor y	≡displaystyle equiv	x∨¯ydisplaystyle xoverline lor y
XOR（异或）	x∨_ydisplaystyle xunderline lor y	≡displaystyle equiv	(x∨¯y)∨¯[(x∨¯x)∨¯(y∨¯y)]displaystyle left(xoverline lor yright)overline lor left[left(xoverline lor xright)overline lor left(yoverline lor yright)right]
XNOR（同或）	x∨_¯ydisplaystyle xoverline underline lor y	≡displaystyle equiv	[(x∨¯y)∨¯x]∨¯[(x∨¯y)∨¯y]displaystyle left[left(xoverline lor yright)overline lor xright]overline lor left[left(xoverline lor yright)overline lor yright]
蕴涵	x→ydisplaystyle xrightarrow y	≡displaystyle equiv	[(x∨¯x)∨¯y]∨¯[(x∨¯x)∨¯y]displaystyle left[left(xoverline lor xright)overline lor yright]overline lor left[left(xoverline lor xright)overline lor yright]
	x←ydisplaystyle xleftarrow y	≡displaystyle equiv	[x∨¯(y∨¯y)]∨¯[x∨¯(y∨¯y)]displaystyle left[xoverline lor left(yoverline lor yright)right]overline lor left[xoverline lor left(yoverline lor yright)right]
等价	x↔ydisplaystyle xleftrightarrow y	≡displaystyle equiv	[(x∨¯y)∨¯x]∨¯[(x∨¯y)∨¯y]displaystyle left[left(xoverline lor yright)overline lor xright]overline lor left[left(xoverline lor yright)overline lor yright]
重言式	Tdisplaystyle mathsf T	≡displaystyle equiv	[(x∨¯x)∨¯x]∨¯[(x∨¯x)∨¯x]displaystyle left[left(xoverline lor xright)overline lor xright]overline lor left[left(xoverline lor xright)overline lor xright]
矛盾式	⊥displaystyle perp	≡displaystyle equiv	(x∨¯x)∨¯xdisplaystyle left(xoverline lor xright)overline lor x

参见

维基共享资源中相关的多媒体资源：或非门

查 论 编 逻辑联结词 恆真（⊤displaystyle top ） 与非（↑displaystyle uparrow ） · 反蕴涵（←displaystyle leftarrow ） · 蕴涵（→displaystyle rightarrow ） · 或（∨displaystyle lor ） 非（¬displaystyle neg ） · 异或（⊕displaystyle oplus ） · 双条件（↔displaystyle leftrightarrow ） · 命题 或非（↓displaystyle downarrow ） · 非蕴涵（↛displaystyle nrightarrow ） · 反非蕴涵（↚displaystyle nleftarrow ） · 与（∧displaystyle land ） 恆假（⊥displaystyle bot ）