njtynhj

正切
性質
奇偶性	奇
定義域	x
到達域	(-∞,∞)
周期	π
特定值
當x=0	0
當x=+∞	N/A
當x=-∞	N/A
最大值	∞
最小值	-∞
其他性質
渐近线	x = (2k + 1)π/2
根	kπ
不動點	0
k是一個整數.

正切（Tangent，tandisplaystyle tan ，东欧国家将其写作tg）是三角函数的一种。它的值域是整个实数集，定义域落在xdisplaystyle xneq kpi +frac pi 2,kin Z。它是周期函数，其最小正周期为πdisplaystyle pi 。正切函数是奇函数。

符号说明

正切的符号为tandisplaystyle tan ，源于英文tangent。该符号最早由数学家T.芬克所采用。

定义

直角三角形中

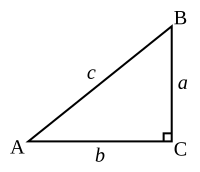
直角三角形，∠C為直角，∠A 的角度為 θdisplaystyle theta ， 對於 ∠A 而言，a為對邊、b為鄰邊、c為斜邊

在直角三角形中，一个锐角的正切定义为它的對邊与鄰邊的比值，也就是：

tan⁡θ=ab=sin⁡θcos⁡θdisplaystyle tan theta =frac textatextb=frac sin theta cos theta ,!

可以發現其定義和餘切函數互為倒數。

直角坐标系中

设αdisplaystyle alpha 是平面直角坐标系xOy中的一个象限角，P(x,y)displaystyle Pleft(x,yright)是角的终边上一点，r=x2+y2>0displaystyle r=sqrt x^2+y^2>0是P到原点O的距离，则α的正切定义为：

tan⁡α=yxdisplaystyle tan alpha =frac yx

单位圆定义

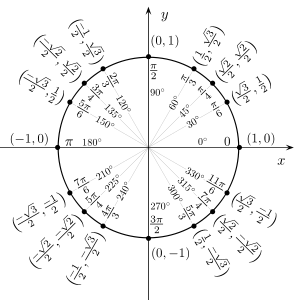
单位圆

图像中给出了用弧度度量的某个公共角。逆时针方向的度量是正角而顺时针的度量是负角。设一个过原点的线，同x轴正半部分得到一个角θ，并与单位圆相交，並令这个交点為y。另原點為O。做一直線，y點，垂直於Oy¯displaystyle overline Oy，並與单位圆相切，令直線與x軸的交點，則此點與y點之距離為正切比值。

单位圆上的正切

单位圆可以被认为是通过改变邻边和对边的长度并保持斜边等于1查看无限数目的三角形的一种方式。

对于大于2πdisplaystyle 2pi 或小于−2πdisplaystyle -2pi 的角度，简单的继续绕单位圆旋转。在这种方式下，有些三角函數变成了周期为2πdisplaystyle 2pi 的周期函数；但由於正切是切線，再绕单位圆旋转時，會出現周期是πdisplaystyle pi ，所以正切是周期为π的周期函数：

tan⁡θ=tan⁡(θ+πk)displaystyle tan theta =tan left(theta +pi kright)

对于任何角度θdisplaystyle theta 和任何整数kdisplaystyle k。

級數定義

正切函數也可以使用泰勒展開式定義

tan⁡x=x+x33+2x515+17x7315+62x92835+...=∑n=1∞B2n4n(4n−1)(2n)!x2n−1displaystyle tan x=x+frac x^33+frac 2x^515+frac 17x^7315+frac 62x^92835+...=sum _n=1^infty frac B_2n4^n(4^n-1)(2n)!x^2n-1

其中B2ndisplaystyle B_2n為伯努利數。

微分方程定义

tandisplaystyle tan 的微分是secdisplaystyle sec 的平方

ddxtan⁡x =sec2⁡xdisplaystyle frac ddxtan x =sec ^2x

另外

∫tan⁡xdx=−ln⁡(cos⁡x)displaystyle int tan x,dx=-ln(cos x)

所以可以用

tan⁡x=(−ln⁡(cos⁡x))′displaystyle tan x=(-ln(cos x))',來定義。

指数定义

tan⁡θ=eiθ−e−iθi(eiθ+e−iθ)displaystyle tan theta =frac e^mathrm i theta -e^-mathrm i theta mathrm i (e^mathrm i theta +e^-mathrm i theta ),

恒等式

用其它三角函数来表示正切

函數	sin	cos	tan	cot	sec	csc
tan⁡θdisplaystyle tan theta	sin⁡θ1−sin2⁡θdisplaystyle frac sin theta sqrt 1-sin ^2theta	1−cos2⁡θcos⁡θdisplaystyle frac sqrt 1-cos ^2theta cos theta	tan⁡θ displaystyle tan theta	1cot⁡θdisplaystyle frac 1cot theta	sec2⁡θ−1displaystyle sqrt sec ^2theta -1	1csc2⁡θ−1displaystyle frac 1sqrt csc ^2theta -1

角的和差

tan⁡(θ±ψ)=tan⁡θ±tan⁡ψ1∓tan⁡θtan⁡ψdisplaystyle tan(theta pm psi )=frac tan theta pm tan psi 1mp tan theta tan psi

正切的有限多项和

设xi=tan⁡(θi)displaystyle x_i=tan(theta _i)，对于i=1,…,ndisplaystyle i=1,ldots ,n。设ekdisplaystyle e_k是变量xidisplaystyle x_i，i=1,…,ndisplaystyle i=1,ldots ,n，k=0,…,ndisplaystyle k=0,ldots ,n的kdisplaystyle k次基本对称多项式。则

tan⁡(θ1+⋯+θn)=e1−e3+e5−⋯e0−e2+e4−⋯,displaystyle tan(theta _1+cdots +theta _n)=frac e_1-e_3+e_5-cdots e_0-e_2+e_4-cdots ,

项的数目依赖于ndisplaystyle n。例如，

tan⁡(θ1+θ2+θ3)=e1−e3e0−e2=(x1+x2+x3) − (x1x2x3)1 − (x1x2+x1x3+x2x3),tan⁡(θ1+θ2+θ3+θ4)=e1−e3e0−e2+e4=(x1+x2+x3+x4) − (x1x2x3+x1x2x4+x1x3x4+x2x3x4)1 − (x1x2+x1x3+x1x4+x2x3+x2x4+x3x4) + (x1x2x3x4),displaystyle beginalignedtan(theta _1+theta _2+theta _3)&=frac e_1-e_3e_0-e_2=frac (x_1+x_2+x_3) - (x_1x_2x_3)1 - (x_1x_2+x_1x_3+x_2x_3),\\tan(theta _1+theta _2+theta _3+theta _4)&=frac e_1-e_3e_0-e_2+e_4\\&=frac (x_1+x_2+x_3+x_4) - (x_1x_2x_3+x_1x_2x_4+x_1x_3x_4+x_2x_3x_4)1 - (x_1x_2+x_1x_3+x_1x_4+x_2x_3+x_2x_4+x_3x_4) + (x_1x_2x_3x_4),endaligned&= "frace_1 - e_3e_0 - e_2 = frac(x_1 + x_2 + x_3) - (x_1 x_2 x_3)"
1 - (x_1 x_2 + x_1 x_3 + x_2 x_3), \ \
tan(theta_1 + theta_2 + theta_3 + theta_4)
&= "frace_1 - e_3e_0 - e_2 + e_4 \ \"
&= "frac(x_1 + x_2 + x_3 + x_4) - (x_1 x_2 x_3 + x_1 x_2 x_4 + x_1 x_3 x_4 + x_2 x_3 x_4)"
1 - (x_1 x_2 + x_1 x_3 + x_1 x_4 + x_2 x_3 + x_2 x_4 + x_3 x_4) + (x_1 x_2 x_3 x_4),endalign "/>

并以此类推。一般情况可通过数学归纳法证明。

半角公式

tan⁡θ2=csc⁡θ−cot⁡θ=±1−cos⁡θ1+cos⁡θ=sin⁡θ1+cos⁡θ=1−cos⁡θsin⁡θ=cos⁡θ+sin⁡θ−1cos⁡θ−sin⁡θ+1displaystyle beginalignedtan frac theta 2&=csc theta -cot theta \&=pm ,sqrt 1-cos theta over 1+cos theta \&=frac sin theta 1+cos theta \&=frac 1-cos theta sin theta \&=frac cos theta +sin theta -1cos theta -sin theta +1endaligned

二倍角

tan⁡2θ=2tan⁡θ1−tan2⁡θ=11−tan⁡θ−11+tan⁡θdisplaystyle beginalignedtan 2theta &=frac 2tan theta 1-tan ^2theta \&=frac 11-tan theta -frac 11+tan theta \endaligned& = "frac11-tantheta-frac11+tantheta\"
endalign"/>

三倍角

tan⁡3θ=3tan⁡θ−tan3⁡θ1−3tan2⁡θdisplaystyle tan 3theta =frac 3tan theta -tan ^3theta 1-3tan ^2theta

正切定理

一个三角形。它的三个内角及其对边。

在平面三角形中，正切定理说明任意两条边的和除以第一条边减第二条边的差所得的商等于这两条边的对角的和的一半的正切除以第一条边对角减第二条边对角的差的一半的正切所得的商。即：

a−ba+b=tanα−β2tanα+β2displaystyle frac a-ba+b=frac mathrm tan ,frac alpha -beta 2mathrm tan ,frac alpha +beta 2"/>

b−cb+c=tanβ−γ2tanβ+γ2displaystyle frac b-cb+c=frac mathrm tan ,frac beta -gamma 2mathrm tan ,frac beta +gamma 2"/>

c−ac+a=tanγ−α2tanγ+α2displaystyle frac c-ac+a=frac mathrm tan ,frac gamma -alpha 2mathrm tan ,frac gamma +alpha 2"/>

用途

物理學

一物體在斜面上剛開始滑動時，其靜摩擦係數為斜面傾角的正切值。

參見

维基共享资源中相关的多媒体资源：正切

• 餘切


• 餘弦


• 正弦


• 正割


• 三角学


• 三角函数

查 论 编 三角函數 基本函數 正弦 · 餘弦 · 正切 · 餘切 · 正割 · 餘割 反函數 反正弦 · 反餘弦 · 反正切 · 反餘切 · 反正割‎ · 反餘割 其他函數 正矢 · 餘矢 · cis函數 · 餘cis函數 · 半正矢 · 半餘矢 · 外正割 · 外餘割 · atan2 · 古德曼函數 定理 正弦定理 · 餘弦定理 · 正切定理 · 餘切定理 · 正割定理 · 勾股定理 其他 三角函數恆等式 · 三角函數精確值 · 三角函数积分表 · 三角函数表 · 雙曲三角函數 · 雙曲三角函數恆等式